Lesson Concept: Josef Albers: Formulation:Articulation
Examining art with a mathematical lens can help students articulate how they see and understand spatial and numerical relationships. Explore prints by Josef Albers to help students expand their understanding of geometric concepts such as parallel lines, congruent angles, rectangular prisms and more.
"I prefer to think that we arrive at insight by experience..."
-Josef Albers, A Conversation with Josef Albers
Josef Albers, artist and teacher
Exploration of the visual perception of color and line predominated the work of Josef Albers from the 1920’s until his death in 1976. Through variation of a theme, Albers challenged the viewer to move away from a conventional visual approach to a new way of seeing and studying color and line relationships.
As a teacher at The Bauhaus (1923-1933), Black Mountain College (1933 – 1949), and Yale (1950-1958), Albers valued student experimentation and discovery – “learning by doing” – over traditional teaching methods.
Thinking about Two and Three Dimensions
As an artist, Albers continually revisited and built upon his own explorations of visual perception. In 1972, at the age of 88, he published Formulation: Articulation, a two-volume set of 122 silk-screened images, each of which was a reformulation of an earlier work. As the preface to the collection states: “The conceptual relationships between color and form inherent in each particular work have been restudied and developed for the medium of silk screening.”
Several of the images, such as the ones below, appear to be revisitations of Albers’ earlier ideas found in his Structural Constellation and Linear Construction series of drawings and prints. In these bodies of work Albers experimented with two-dimensional renderings of three-dimensional floating forms which a viewer might perceive in multiple positions and from shifting perspectives. This perceptual ambiguity makes these artworks wonderful playgrounds for exploring geometric concepts.
Formulation - Articulation I
Formulation - Articulation II
Formulation - Articulation II
Formulation - Articulation II
Re-Presentation Challenge
Just as Albers revisited ideas from earlier in his career by “restudying” them with another medium, students may find insight with a similar task. Tailor this simple, open-ended activity for your own students.
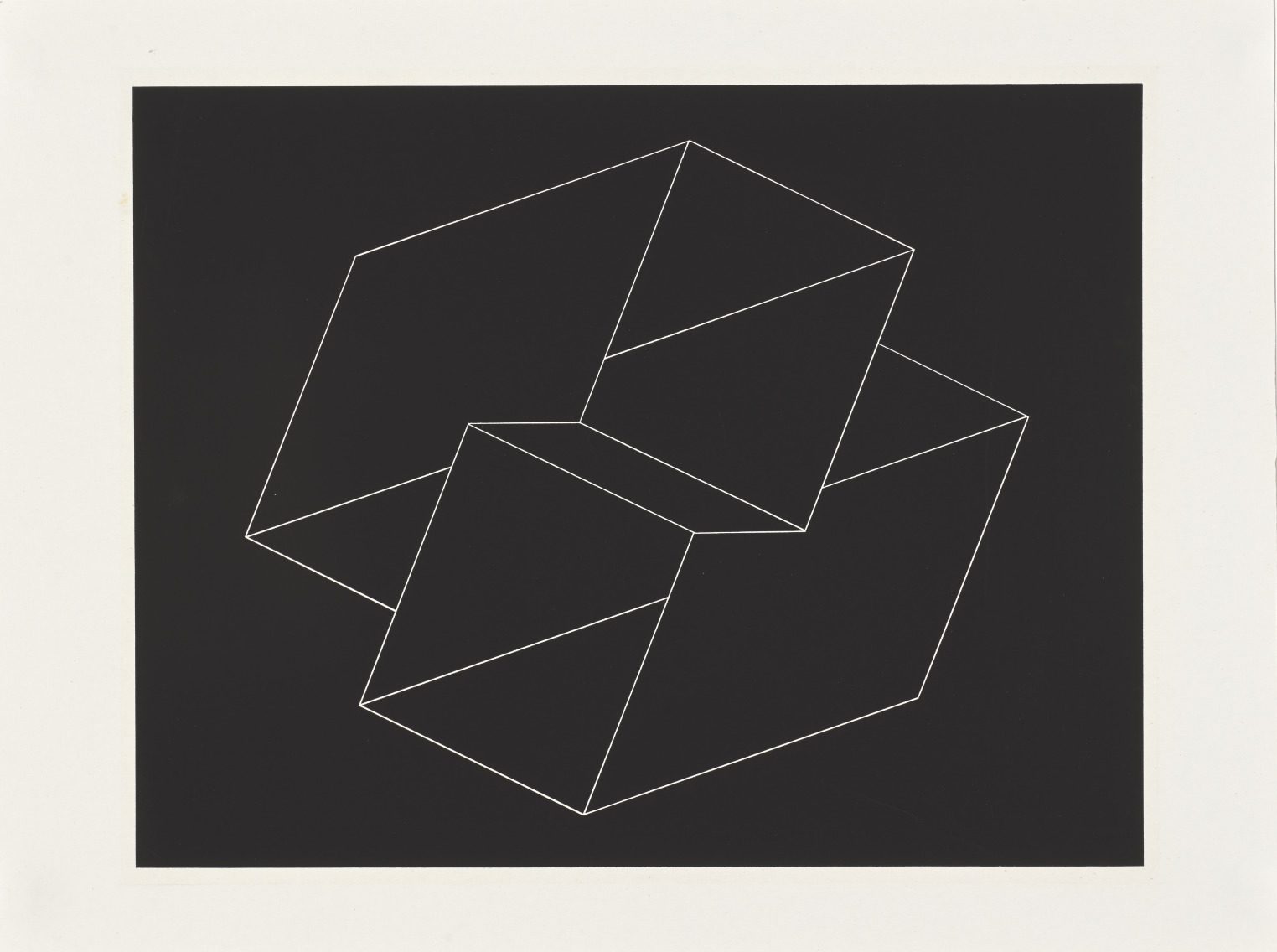
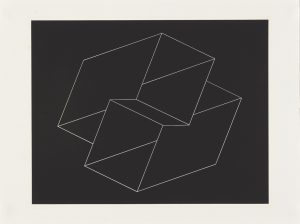
Ask them to explore this Albers image from Folio II of Formulation:Articulation using both two-dimensional and three-dimension tools.
Challenge students to look carefully at the image. Could this object possibly exist in three-dimensional space? Try to reproduce or represent the Albers’ structure with one or all of these simple tools:
- Pencil and paper. Can you redraw this simple image?
- Scissors and paper. Can you fold, and/or cut paper to reproduce the structure?
- Play-dough or modeling clay. Can you see a way to build the form as a three-dimensional structure?
There is no one correct way to accomplish the task. The goal is to THINK about and GRAPPLE with geometric relationships rather than neatly define what they are.
What's the Big Idea? Talking it Through
Come back as a group and share observations.
- Did students make any discoveries about the structure that they had not initially noticed?
- What was difficult or easy about this task? How did they organize their thoughts in order to break down the problem and proceed with the task?
- Looking at this challenge through a mathematical lens, what ideas did they have? What spatial and linear relationships did they discover? What vocabulary did they use to articulate these ideas?
Make a class list of student observations that address Geometry concepts you may be introducing for study. Consider the vocabulary, both formal and informal, students are using to talk about these concepts. Use their ideas as a reference and springboard for more formal instruction and problem-solving.
Select Bibliography
Albers, Josef. Interaction of Color. London: Yale, 1979. Unabridged text and selected plates. Revised Edition.
Cossey, Ruth., and David M. Donahue. “Mathematics:Logic and Imagination.” In Art-Centered LearningAcross the Curriculum, edited by David M. Donahue and Julia Marshall,141-160. New York, NY:Teachers College Press, 2014.
Danilowitz, Brenda, and Nicholas Fox. Weber. The Prints of Josef Albers: A Catalogue Raisonne, 1915-1976. New York: Hudson Hills Press, 2009.
Holloway, John H., and John A. Weil, and Josef Albers. “A Conversation with Josef Albers.” Leonardo 3,No. 4 (1970); 459-64.
Horowitz, Frederick A. “What Josef Albers Taught at Black Mountain College, and What Black Mountain College Taught Josef Albers.” Journal of Black Mountain College Studies 1 (Spring) 2011 http://www.blackmountainstudiesjournal.org/volume-11-spring-2011/1-9-frederick-a-horowitz/
“Josef and Anni Albers Foundation” Josef and Anni Albers Foundation. https://albersfoundation.org.
Text panel copy for Josef Albers Formulation: Articulation. 1992. TS, Exhibition Files, Virginia Museum of Fine Arts Library, Richmond.
Weber, Nicholas Fox. Josef Albers: A Retrospective:. New York: Solomon R. Guggenheim Museum, 1988.
